Difference between revisions of "Heat transfer"
| Line 21: | Line 21: | ||
**velocity of the fluid (v) | **velocity of the fluid (v) | ||
**thermal gradient (∇T) | **thermal gradient (∇T) | ||
| + | |||
| Line 63: | Line 64: | ||
At some critical Rayleigh (Racr) number (dependent on the system), the convection regime shifts. | At some critical Rayleigh (Racr) number (dependent on the system), the convection regime shifts. | ||
| + | |||
| + | |||
| + | == Indoor Natural Convection and Different Rayleigh Numbers== | ||
| + | *I'm | ||
| + | *making a list | ||
| + | **it's here | ||
=='''Heat Transfer in Ice Stream Shut Down and Start (based on Joughin and Alley, 2011)'''== | =='''Heat Transfer in Ice Stream Shut Down and Start (based on Joughin and Alley, 2011)'''== | ||
Revision as of 00:36, 13 March 2019
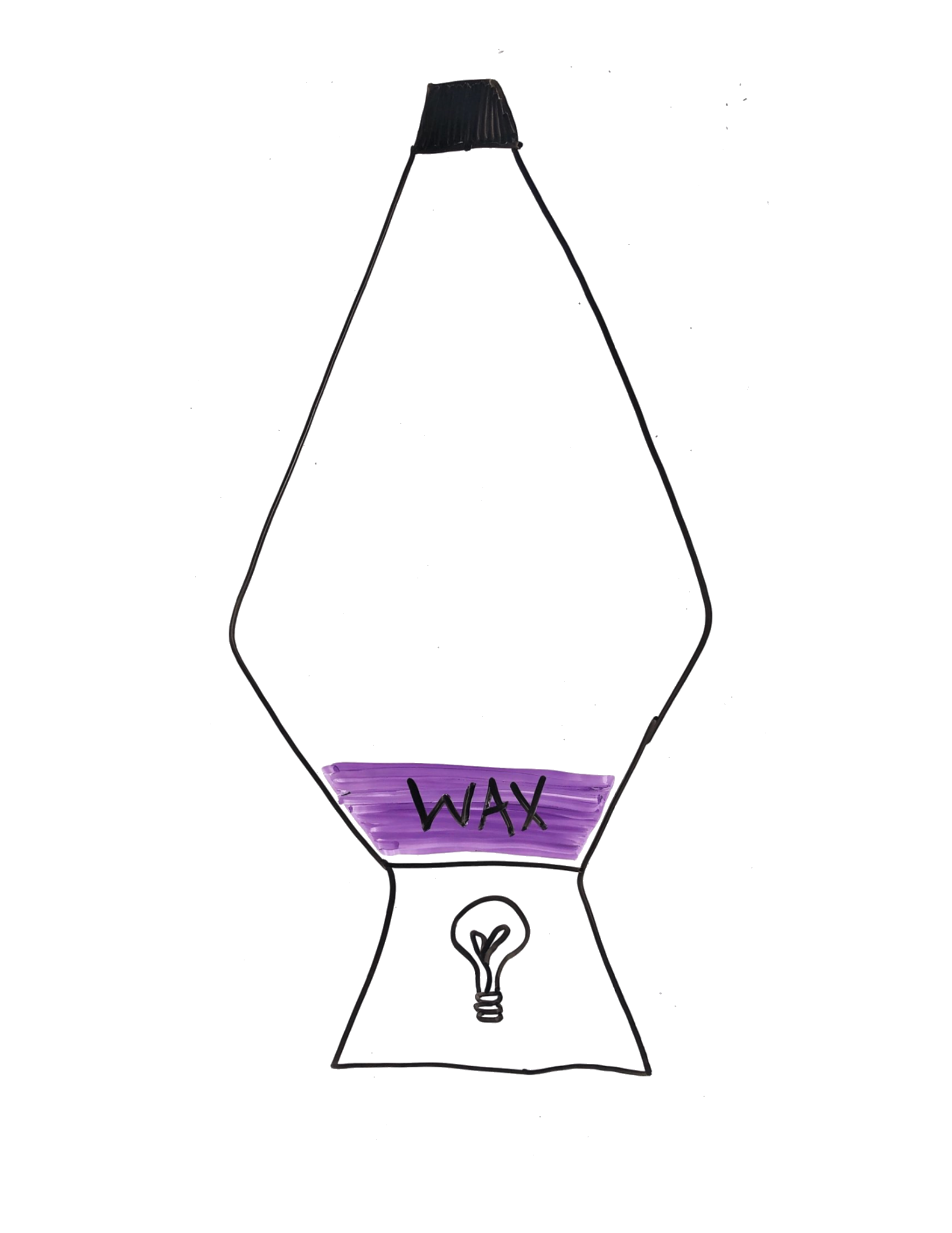
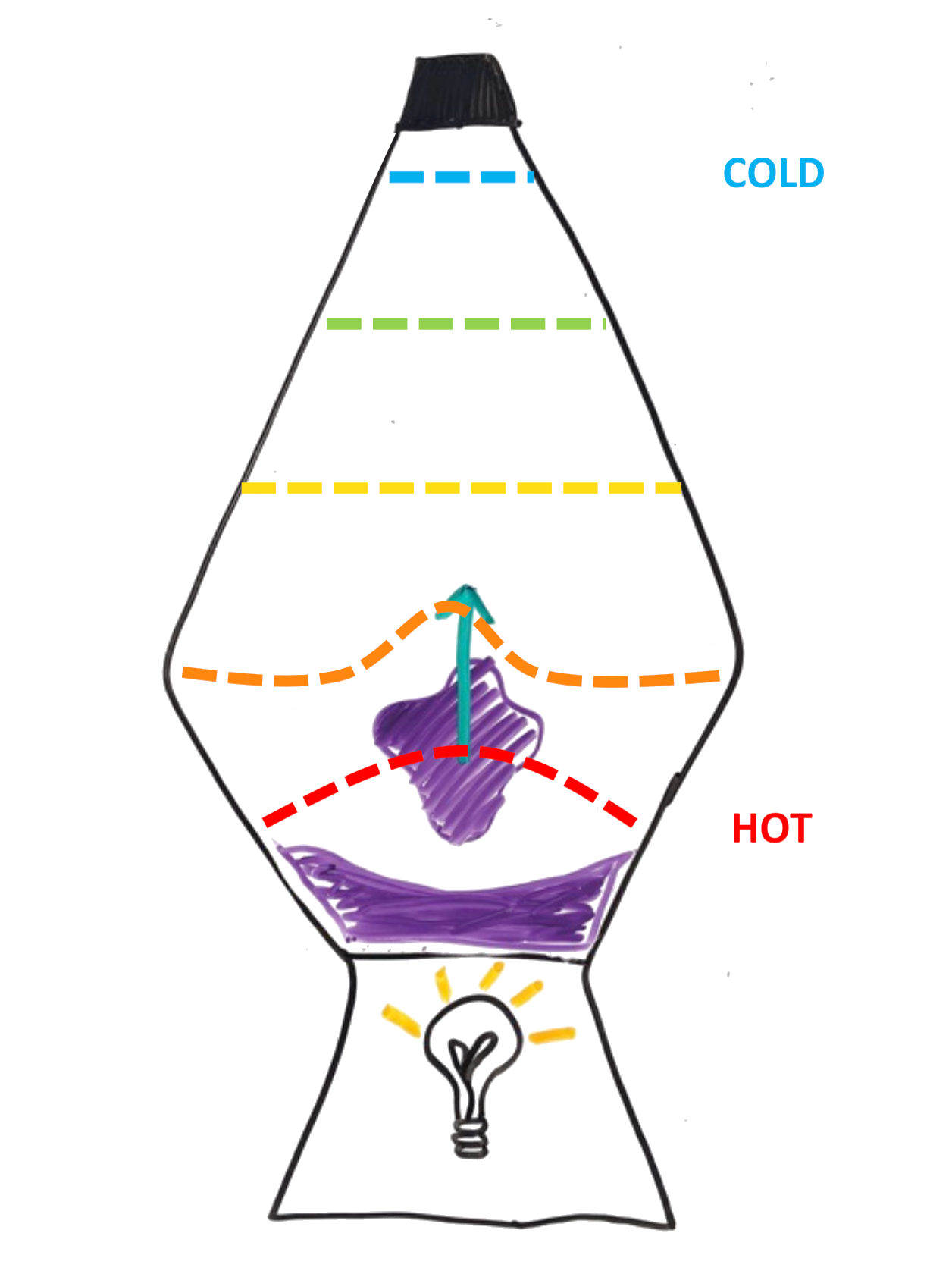
Heat Transfer in a Lava Lamp
When you flip on the light in a lava lamp, heat from the bulb works slowly to warm up the wax (or ‘lava’). This kind of heat transfer is called conduction, or movement of heat from one place to another. Heat energy flows from high heat (light bulb) to low heat (wax), and the gradient is the driver of conductive heat transfer.
Until things start moving, conduction is the dominant heat transfer process in our lava lamp system.
- Conduction, defined as κ∇²T depends on:
- thermal diffusivity of the material through which heat is moving (κ)
- thermal gradient (∇²T)
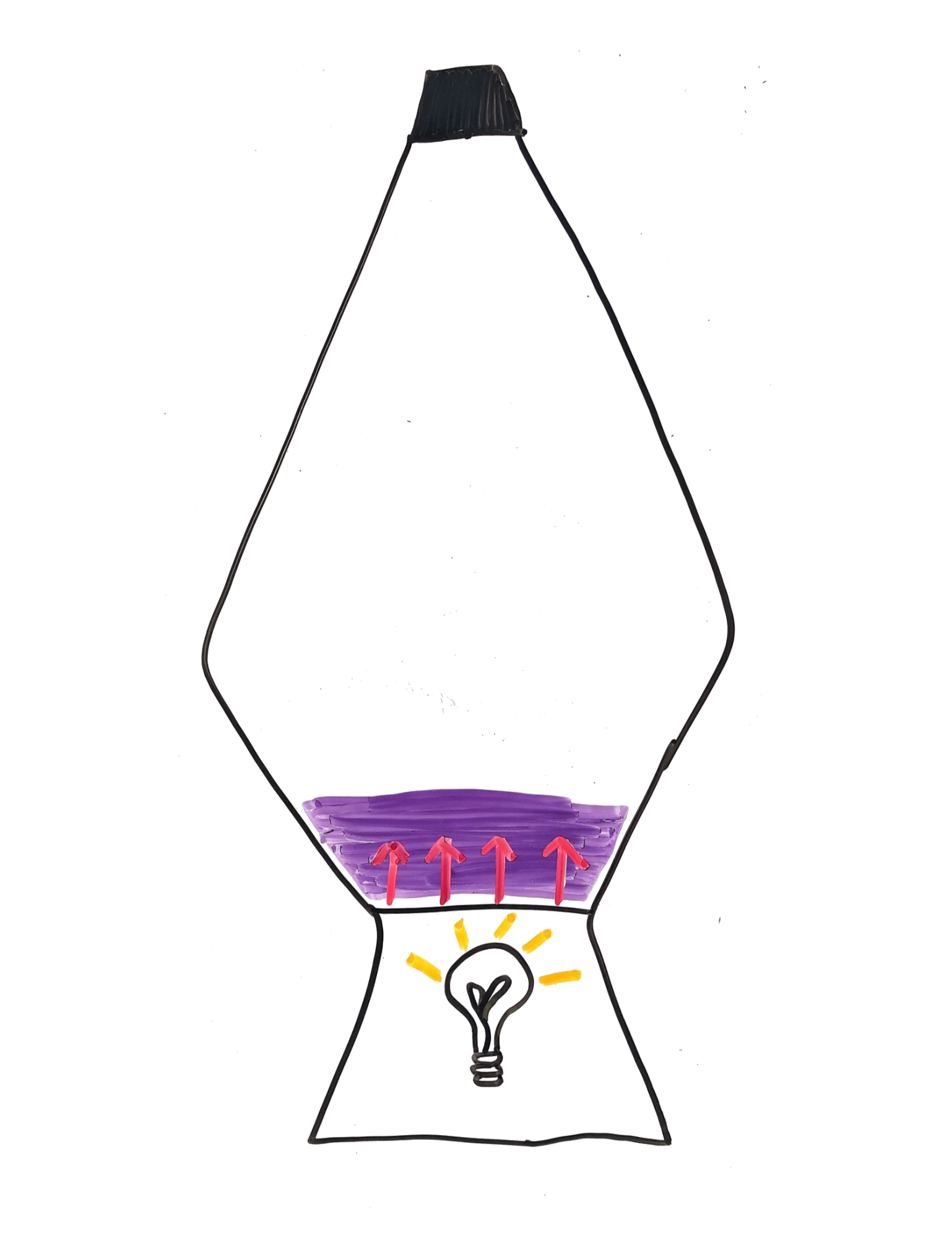
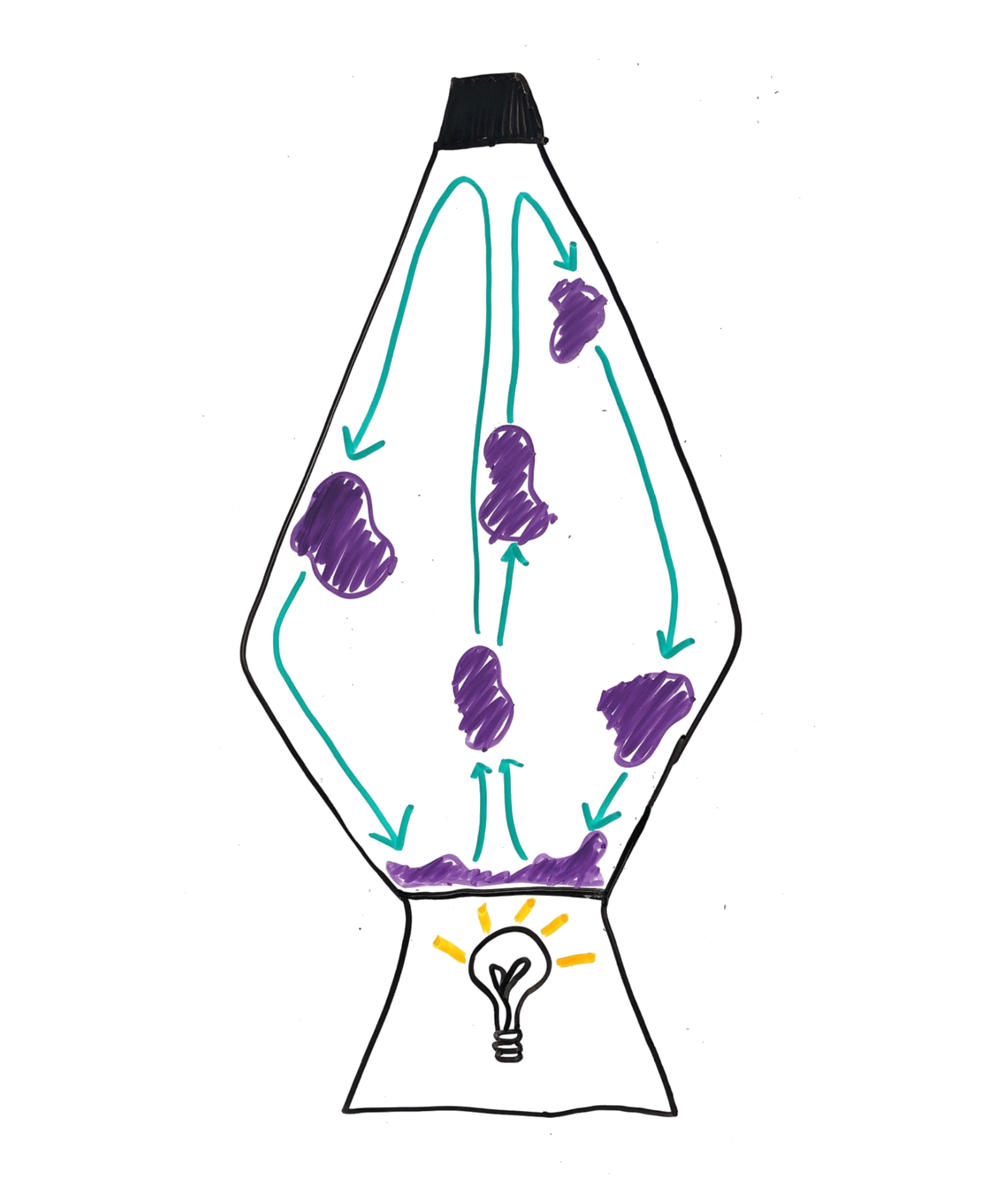
The density of the wax is inversely related to temperature, meaning that at higher temperatures, the wax is less dense. When the density becomes lower than the density of the liquid in the lava lamp, the wax will tend to float upward.
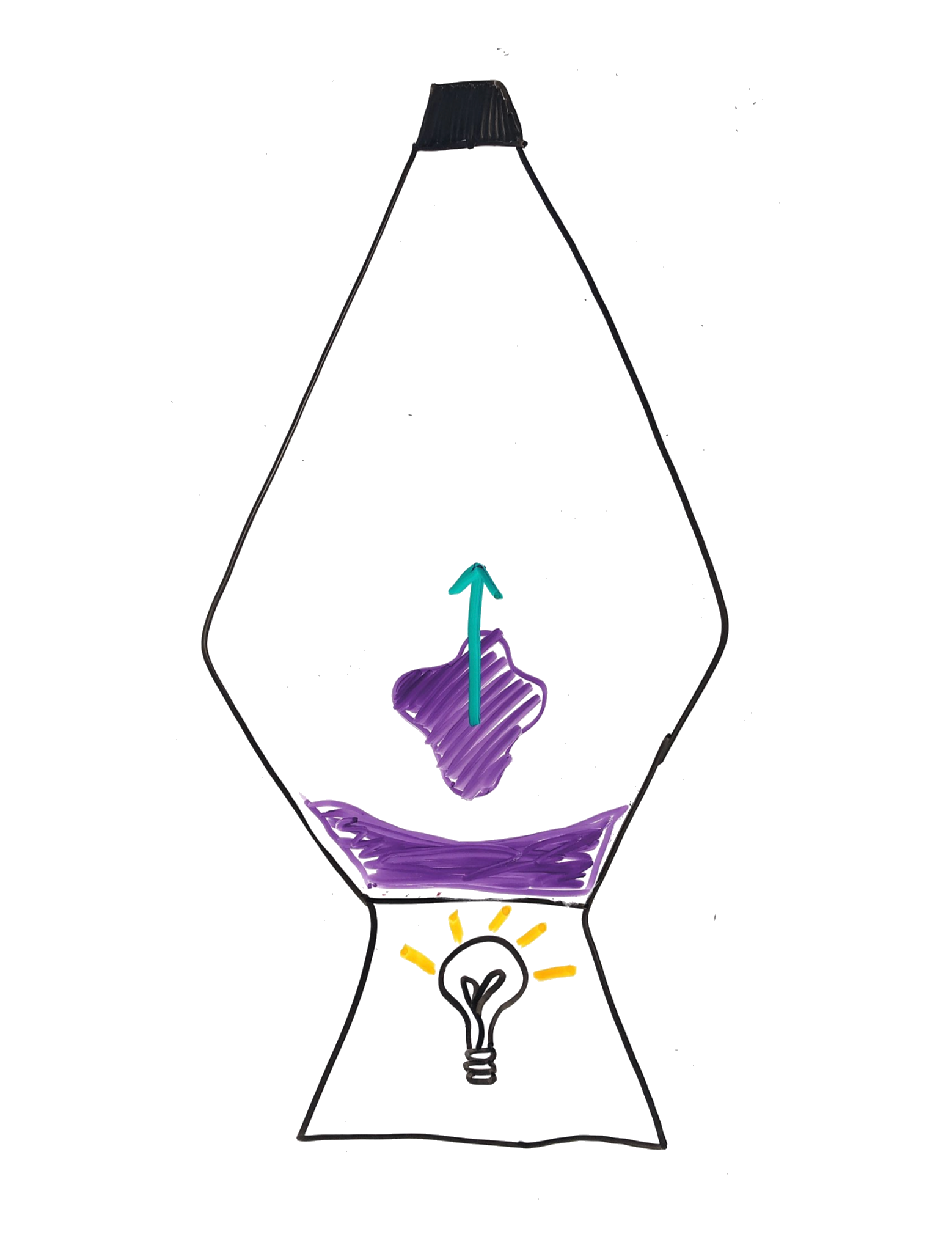
Wax reaches critical density (less than liquid) and tends to float upward. That movement of wax, and thus heat, introduces velocity into the system and is a process that is referred to as advection.
- Advection, defined as v∇T depends on:
- velocity of the fluid (v)
- thermal gradient (∇T)
Heat Transfer Equation:
Heat transfer within the lava lamp can be represented by an equation. The heat transfer equation brings these aforementioned processes together to describe how heat changes through time (∂T/∂t). It is defined as:
∂T/∂t = κ∇²T + v∇T + A
∂T/∂t = conduction + advection + production
The production term (A) represents internally generated heat energy and we do not consider it in this lava lamp scenario. It becomes important in systems such as glaciers, when internal strain heating is an important source of heat.
Peclét Number:
The Peclét Number is a dimensionless number which indicates whether conduction or advection dominates the system.
Pe = uL/κ
where u = flow velocity, L = characteristic length Height of lava lamp, κ = thermal diffusivity
- Low peclet regime- dominated by heat transport by conduction
- High peclet regime- dominated by heat transport by advection
With the movement of wax in the upward direction away from the heat source at the bottom of the container and into a cooler material the wax density increases again (more than liquid). This wax density increase causes the wax to sink back down to the bottom of the container towards the heat source. This process is called convection.
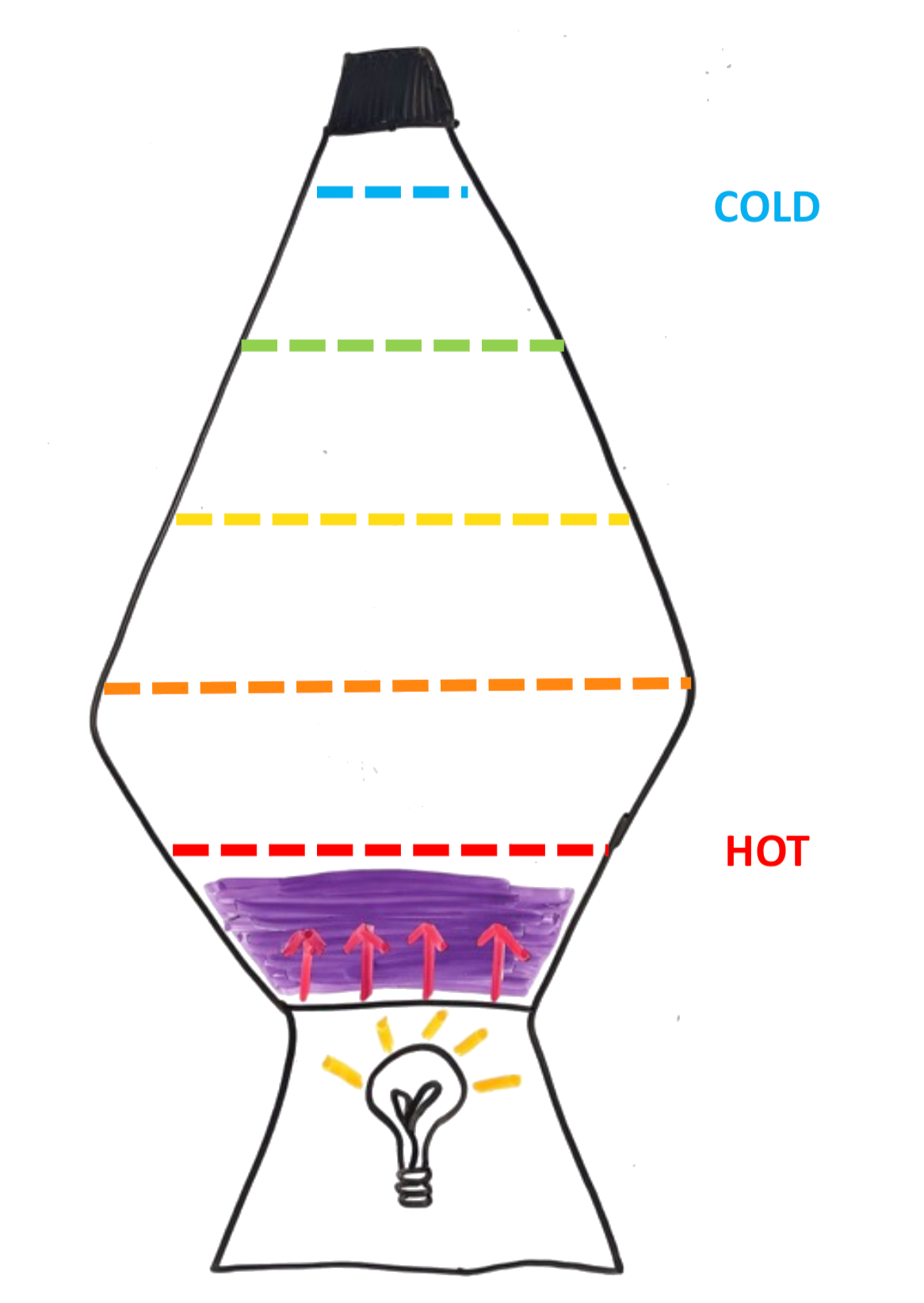
Rayleigh Number:
The Rayleigh Number is a dimensionless number used to describe whether convection grows or decays.
Ra ≡ (gρΔTαd³)/μκ
- Convection depends on driving forces and resisting forces.
- Driving forces (when these terms are dominant convection grows):
- Acceleration due to gravity (g)
- Density (ρ)
- The temperature difference between the bottom and top of the convection cell (ΔT)
- The volume coefficient of thermal expansion (α)
- The height of the convection cell (d)
- Resisting forces (when these terms are dominant convection decays):
- Viscosity (μ)
- Thermal diffusivity (κ)
- Driving forces (when these terms are dominant convection grows):
At some critical Rayleigh (Racr) number (dependent on the system), the convection regime shifts.
Indoor Natural Convection and Different Rayleigh Numbers
- I'm
- making a list
- it's here
Heat Transfer in Ice Stream Shut Down and Start (based on Joughin and Alley, 2011)
Stop and start of ice streams is dependent on:
- Thinning (steepens basal temperature gradients)
- Basal freezing (puts on the brakes for a system)
- Thickening (build up traps geothermal heat)
- Ice stream activation & the cycle is repeated
Watch the video below to walk through the steps of ice stream shut-down and speed-up, and see how this relates to the heat transfer equation introduced above.
Further reading:
Different theories exist for ice stream shut down, and here we present varying hypothesis. Please note that the behavior we model in our video is based upon the hypothesis of Joughin and Alley (2011).
- Joughin, I., and R. B. Alley (2011) Stability of the West Antarctic ice sheet in a warming world. Nature Geosci., 4, pp. 506–513. https://www.uib.no/sites/w3.uib.no/files/attachments/joughinnatgeoreview2011.pdf
- Anandakrishnan, S., Alley, R.B., Jacobel, R.W. and Conway, H. (2001) The flow regime of Ice Stream C and hypotheses concerning its recent stagnation. The West Antarctic Ice Sheet: Behavior and Environment, AGU Antarctic Research Series, 77, pp. 283-296. https://www.researchgate.net/profile/H_Conway/publication/266340045_The_Flow_Regime_of_Ice_Stream_C_and_Hypotheses_Concerning_Its_Recent_Stagnation/links/54f92db90cf210398e979b18.pdf
- Catania, G.A., Scambos, T.A., Conway, H. and Raymond, C.F. (2006) Sequential stagnation of Kamb ice stream, West Antarctica. Geophysical Research Letters, 33(14). https://doi.org/10.1029/2006GL026430
- Hulbe, C. & Fahnestock, M. (2007) Century-scale discharge stagnation and reactivation of the Ross ice streams, West Antarctica. J. Geophys. Res.-Earth 112, https://agupubs.onlinelibrary.wiley.com/doi/pdf/10.1029/2006JF000603
- Lipovsky, B., and E. Dunham (2016) Tremor during ice-stream stick slip, Cryosphere, 10(1), pp. 385–399. https://www.the-cryosphere.net/10/385/2016/tc-10-385-2016.html
- Price, S.F., Bindschadler, R.A., Hulbe, C.L. and Joughin, I.R. (2001) Post-stagnation behavior in the upstream regions of Ice Stream C, West Antarctica. Journal of Glaciology, 47(157), pp. 283-294. https://doi.org/10.3189/172756501781832232